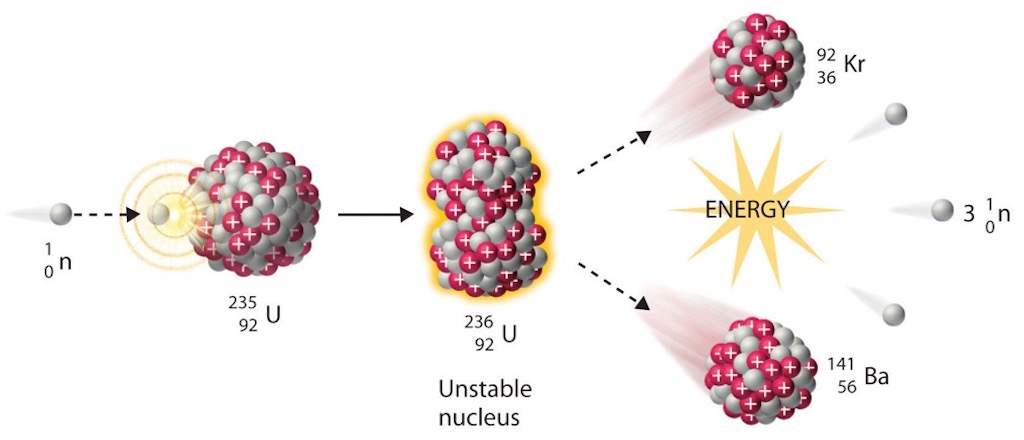
At 3:20 p.m. on December 2, 1942, Fermi’s massive lattice pile of 400 tons of graphite, six tons of uranium metal, and fifty tons of uranium oxide achieved the first self-sustaining chain reaction, operating initially at a power level of one-half watt (increased to 200 watts ten days later).
Above is the only original photograph of the reactor being built.
As Compton reported to Conant, “the Italian navigator has just landed in the new world”. To Conant’s question, “Were the natives friendly?” Compton answered, “Everyone landed safe and happy”. Significant as this moment was in the history of physics, it had already been decided to move to the pilot plant and Groves had already instructed Du Pont to move into design and construction on December 1, 1942.
For the test, most people were on the balcony of the court. Norman Hilberry, equipped with an axe, was prepared to sever a rope tied to a balcony rail. This would drop into place an emergency safety rod suspended over the pile (Hilberry went on to direct the Argonne National Laboratory). George Leon Weil handled the final control rod. On the platform above the pile, three men stood ready to flood it with cadmium salt solution, which would absorb sufficient neutrons to halt a runaway reaction (they were called ‘the suicide squad’). Also in the control room they had command of the safety rods. As the last control rod was removed, foot-by-foot, tests were performed. Halfway through Fermi said he was hungry, and they broke for a lunch lasting a little more than two and one-half hours. They continued removing the control rod shortly after 14:00, and declared that the reaction was self-sustaining at 15.25. Wine from a now famous flask of Chianti was poured into a paper cup and passed around. No toast, no remarks, nothing.
On December 28, 1942, Roosevelt approved an investment of $500 million to build full-scale gaseous-diffusion and plutonium plants and the compromise electro-magnetic plant (smaller than the others), as well as heavy-water production facilities.
The procurement and engineering functions of the Planning Board were taken over by the Manhattan District in the summer of 1942 and by the spring of 1943 the Manhattan District took over the research and development contracts from the OSRD. The transfer was made on May 1, 1943, and marked the end of the formal connection of OSRD with the uranium project.
In many way the Manhattan Engineering District had become a big construction project. It purchased and prepared sites, let contracts, hired personnel and subcontractors, built and maintained housing and service facilities, placed orders for materials, developed administrative and accounting procedures, and established communications networks.
By the end of the war Groves and his staff had spent approximately $2.2 billion on production facilities and towns built in the states of Tennessee, Washington, and New Mexico, as well as on research in university laboratories from Columbia to Berkeley.
What made the Manhattan Project unlike other companies performing similar functions was that, because of the necessity of moving quickly, it invested hundreds of millions of dollars in unproven and hitherto unknown processes and did so entirely in secret. Inherent to this approach was what is now often called trial-and-learning experimentation, a way to mix problem solving and innovation. Also called ‘experimentation in the unknown’, the focus was (and still is today for many companies) on inventing something while dealing with incomplete knowledge or even profound ignorance. This type of experimentation is often used when there are several different problems to solve, when there are many alternatives to test, and when the evaluation criteria are not pre-given. When the Manhattan Project started the fundamental principles were clear, but theories were full of unverified assumptions. In some cases no theories were available, so experimentation (trial and error) was the only solution. Groves called it “proceeding in the dark”. For example, the work on electro-magnetic separation was all about magnetic shims, sources, and collectors that gave the best results, although no one could explain why one combination was better than another. Perhaps the most obvious example was the development of the implosion technique. The starting point, in July 1943, were incredibly crude experiments using TNT surrounding hollow steel cylinders. It was John Von Neumann, in September 1943, that suggested arranging shaped charges in a spherical configuration around the active material, and aim for a faster kind of implosion based upon increasing the amount of high explosive. The final assembly consisted of a 5-foot diameter sphere composed of ‘lens’ for a total of nearly 3 tons of high explosive. The theory work only started in January 1944, with the analysis of Edward Teller on the (shock wave) hydrodynamics of implosions. Calculations were impossible by hand, and were at the limit of the computing power available at that time. This was one of the reasons why Los Alamos became a pioneer in using computers (they received a big IBM machine in April 1944). In 1944 there were analytic solutions for shock waves in perfect gases, but nothing for nuclear materials in a temperature-pressure-energy region of interest in an atomic bomb. Despite that, Teller was able to show that an implosion was possible, and he was able to define the kinds of experiments needed. The impact of these initial calculations and experiments was that two new divisions, ‘Gadget’ G Division and Explosives X Division, were created. Throughout the first half of 1944 the focus was on how to measure (extremely fast) implosion parameters such as symmetry, time of collapse, and degree of compression. They ended up identifying seven different approaches, ranging from X-ray photography, through the RaLa method, to a so-called betatron method. For example, the X-ray photography revealed ‘jet’ formations during implosion, and at the same time pushed the development of very precise timing circuits. In this particular example, the RaLa method, invented by Robert Serber in November 1943, involved placing a gamma-ray source in the centre of the spherical assembly. The gamma-rays would travel out radially, and where affected by the density changes in the collapsing sphere of metal. The data provided time of collapse, and degree of symmetry and compression. Nice idea, but they then had to find a good gamma-source (so-called RadioLantanum-140) and design new ionisation chambers (that would be destroyed in the explosion), new recording systems, and build a test site with a bomb-proof shelter. Just getting RadioLantanum-140 required Oak Ridge to build a special extraction laboratory and a second plant for dissolving irradiated uranium slugs and recovering barium from the solution (and then secretly ship it 1,200 miles to Los Alamos). RadioLantanum-140 was the most powerful radiation source people had worked with at that time. Experiments started September 22, 1944, and only on December 14, 1944 did it really work properly. Electronic detonators appeared in February 1945, along with the first observation of sizeable compression. And by June 1945, they had a viable design for the explosive ‘lenses’. Some experts have summarised this entire process as “blowing things up and looking to see what happened”. There was no established art for implosion devices. Experiments were the only option with no ‘a priori‘ knowledge. And each experiment lead to new questions and new problems, which lead to new multi-disciplinary groups being formed. And each experiment required a new generation of measurement instruments (the experiments were not possible with existing technology). A key (hidden) concept seen in this example, was to tell the difference between failures and mistakes. Mistakes were wrong experiments that did not help in the understanding of a problem, failures were to be learned from and pointed to improvements needed. The reality was that the implosion example had both, so it was important to understand the difference and learn (in different ways) from both. In some cases there was no way to tell the difference between a mistake and a failure, so the key was to “amass a body of understanding” through a series of overlapping experiments, none of which would individually have been able to provide the solution.
One nice ‘general’ rule that has been identified is that if you are really looking at a problem that requires radical innovation, then the available instruments of measurement and test are almost always inadequate, or even inexistent.
For the Manhattan Project the need for haste clarified priorities and shaped decision making. Unfinished research on three separate, unproven processes had to be used to freeze design plans for production facilities, even though it was recognised that later findings inevitably would dictate changes. The pilot plant stage was eliminated entirely, violating all manufacturing practices and leading to intermittent shutdowns and endless troubleshooting during trial runs in the production facilities.
In July 1943 Conant and Richard Chace Tolman were formally asked by General Groves to serve as his scientific advisers. They had already been doing so informally and continued to do so. Coordination of the various scientific and technical programs was accomplished by meetings between General Groves and the leaders of the various projects, in particular, Compton, Lawrence, Oppenheimer, and Urey.
The Physics of the Pile
You are going to have to still live with a multitude of terms describing the same thing, e.g. pile, core, and reactor all mean the same thing, and uranium usually meant natural uranium oxide, and metal often meant uranium metal, etc. And of course the wonderfully evocative ‘lumps’ was just a term used to describe the uranium blocks or units that were the fuel for the pile.
We are going to try to delve into the physics of pile as it evolved. We will start with what looks to be a really secondary problem. In early 1942 the question arose, if a pile generated heat, would it be more reactive or less? For safety reasons it would be better that the pile had a negative temperature coefficient (for a short period the Chernobyl reactor had a positive temperature coefficient and we all know what that meant). In simple terms a negative temperature coefficient means that if the pile’s operating temperature is exceeded the reactivity is reduced. In fact the temperature coefficient is one of the most important physical quantities that defines the inherent stability of a reactor. Important, but difficult to calculate, and so it is often something that is measured in critical assemblies (such as the Chicago pile).
What can happen when the temperature in a reactor increases? Firstly, the resonance absorption increases due to the broadening of the resonance levels. Secondly, the fraction of thermal neutrons lost by absorption in the moderator decreases. Thirdly, the diffusion length of thermal neutrons increases, and so does loss through leakage outside the pile. This third factor is dependent upon the physical geometry of the pile.
Femi concluded that for a critical pile, an increase in temperature of 700°C would produce a small increase in the multiplication factor, nevertheless when the first pile was built the temperature coefficient was one of the first things Fermi wanted to measure.
As we continue to follow Fermi, we have to move to Chicago, and we will see the idea of a large-scale pile experiment become a reality.
In Chicago one of Fermi’s ‘new’ collaborations was with Gregory Breit on the topic of reflectors and what they called a ‘seed’ or core. The starting point was that a natural uranium and graphite system was not likely to have a very high multiplication factor ƙ, more than 1.0, but not high. Things were expected to be better with uranium metal and a purer form of graphite. One idea was to concentrate the higher quality graphite in the ‘core’ and surround the core with some other ‘neutron regenerative structure’ having a lower multiplication factor. It turned out that using a reflector reduced considerably the amount of enriched uranium needed. In addition it was found that there would be more neutrons in the reflector than in the core or ‘seed’, so that the main production of plutonium-239 could take place in the reflector.
The volume needed for a chain reaction with a seed but without a reflector was about 180 tons, consisting of about 32 tons of natural uranium oxide. With a seed and a reflector the amount of natural uranium oxide needed was reduced by between ⅛ and ⅓ of the amount needed without a reflector, their best estimate was a requirement of 11-12 tons instead of 32 tons.
As Fermi waited for the arrival of sufficient material for his first chain reaction, he continued to improve his understanding of a variety of physical constants. His interests ranged over absorption cross-sections for thermal neutrons in both uranium oxide and graphite, the effect on neutron leakage due to the presence of many empty ducts through the pile, the effect of temperature changes on the multiplication factor ƙ, and the nuclear properties of various materials likely to appear in a industrial pile.
Also during this time the exponential pile was constantly being built, modified, and re-built, depending upon the latest experiments. One particular series of tests showed convincingly that the latest delivery of ‘pure’ uranium oxide was truly chemically pure, and that the uranium oxide used a year ago in Columbia had been “filthy”. Fermi found a multiplication factor ƙ of 1.04 for exponential pile No. 9 (i.e. the 9th re-build of the same basic components).
In Chicago Fermi repeated an experiment he had conducted in Columbia, the measurement of the number of neutrons emitted by uranium per thermal neutron absorbed 𝜼. In fact in Chicago Fermi could direct a team to perform all the experiments he wanted, and even have the results analysed by team of theoreticians. In this case he did the analysis himself. The issue was to use an experimental set-up with a better geometry and with purer materials. The results Fermi obtained was 𝜼 = 1.29, which was significantly lower than the figure he had found at Columbia. All the more reason to check and re-check everything.
According to Fermi’s notes the site just outside Chicago was originally bought in June 1942 to house the future reactors for producing element-94 (plutonium). The Army quickly realised that the site was not big enough, so the Oak Ridge site was purchased. The so-called ‘Argonne Forest’ site was freed for everything but the construction of the first production reactors. So it was there that the team decided to build the experimental pile. Eventually the plans would change again and the pile would be built on the university campus.
In September 1942 the weekly reports started to include a section for the preparatory work for the experimental pile. The first experiment that I’ve come across was about the idea to enclose the pile in a rubberised balloon cloth which would allow the pile to be evacuated of air (in the end the core was not evacuated). Tests were also done on cooling the granite pile with water running through copper pipes embedded in the outer portion of the graphite. They also tested the idea to fill the evacuated balloon cloth with helium. In each case they studied the energy dissipation per unit area under ‘operational’ conditions. Work also progressed on the physics of the four control rods.
From October 1942 the focus on the experimental pile increased (still planned to be built in the Argonne Forest). It all started by pressing about 50 tons of uranium oxide and machining about 500 tons of graphite. This work was done sitting under the ‘famous’ West Stand. Over time the general idea of the pile evolved. In October it had become a spherical structure of about 9 m in diameter with a central lattice holding lumps of about 2 kg each. In parallel work continued with exponential experiments with uranium metal lumps.
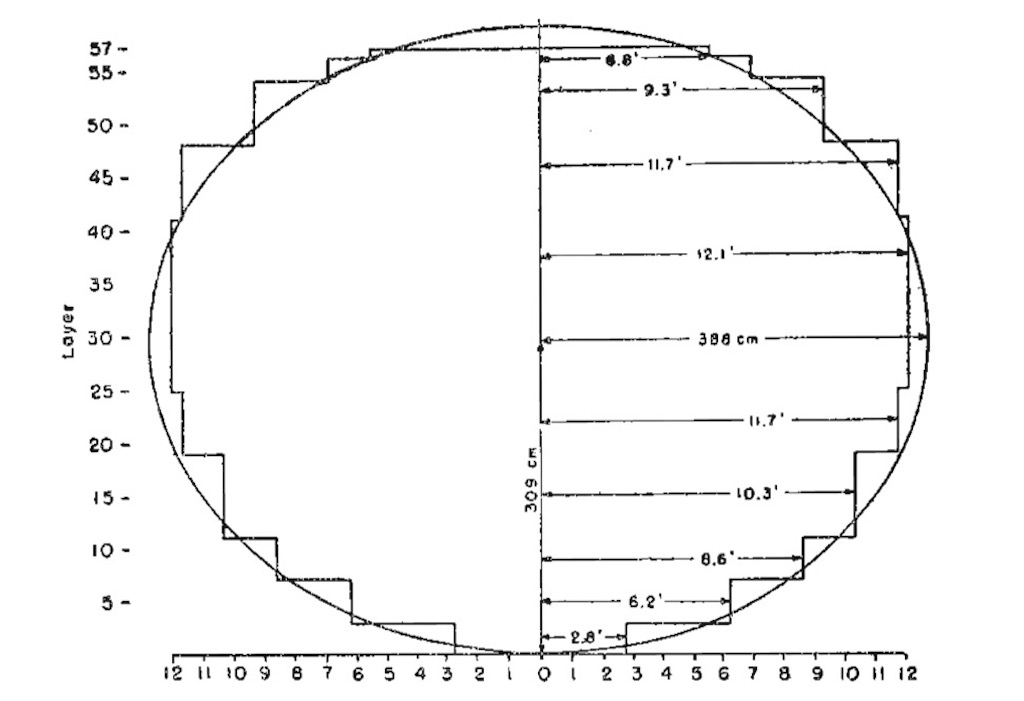
What we see above is a lattice of lumps, partly of uranium oxide and partly uranium metal embedded in graphite. The entire pile was made up of cubes of 21 cm on a side. About 6 tons of metal was available so the idea was to build a pile of approximately spherical shape using the best graphite and uranium metal in the centre. The pressed uranium oxide lumps weighted about 2.1 kg each. Finally it did not work out as a real sphere, but it was 309 cm top-to-bottom and 388 cm across the equator. There was a wooden structure that kept it all together. The calculations for this design put the multiplication factor ƙ at 1.039.
More or less in parallel with the work on the Chicago pile Fermi chaired another team looked at how to build a reactor for producing plutonium. One of the first issues was the choice of a cooling system with a sufficiently low neutron absorption. There were lots of ideas, ranging from liquid bismuth, through liquid uranium hexafluoride, to uranium oxide as a liquid slurry. It was clear that to produce plutonium the reactor(s) would need to dissipate 100’s of megawatts of heat. And corrosion and radiation shielding would also be additional problems. The real challenge was that the best engineers had never even heard of a neutron!
Naturally Fermi also studied some of his own ideas for cooling. The first was with a system of water pipes passing through the graphite structure and the core of uranium fuel. The heat from the lumps must be conducted through the graphite to the water tubes. The other alternative was to put the uranium metal in cylinders (rods) packed together and running through the graphite, and then to run a single water tube though the middle of these cylinders. In some ways one was cooling the graphite and the other cooling the fuel. Fermi weighted up the pro’s and con’s of the two options. The water pipes fitted in with the way the core design had been modelled, so the neutron properties were well known. The small number of cooling pipes would have a limited impact of the reactivity of the pile. The down side was that the clearances between the graphite, pipes and lumps must be small, and there may be problems with thermal distortion of the pile. Also it would be difficult to disassemble the pile after the tests. Concerning the second idea it would be easy to disassemble the core, and the fuel would be easy to handle and reprocess. The construction would be easy, and no particular precision would be needed. The down-side was the large loss in reactivity, and the uranium would need to be prepared in ‘shots’ (what we would call pellets today). Fermi’s conclusion was that both ideas were not perfect, and perhaps surface cooling of the pile was the better idea. This might mean running the reactors at a lower output, but might make the reactors easier to operate.
In November 1942 most the basic material for the pile had arrived, and the task at hand was to start building the pile. But there was a strike of the labour unions, so Fermi simply proposed to build the pile in the West Stand, even if it would have to run at nominal power. Compton gave Fermi the go-ahead, and they started building on November 14, 1942.
It’s impressive to note that on the one hand Fermi was starting to plan the first test of the pile, and on the November 18, 1942 Du Pont had accepted to build large scale piles for plutonium production at the Hanford site.
Whilst the decision to build the pile in the West Stand dates from November 14, 1942, in fact Fermi had been building the pile there from early October. As the graphite arrived the bars were machined to size and holes were drilled to accommodate the uranium lumps. The machines used were normal wood-working machines, and some of the bricks were drilled with a 8.35 cm hole to take the uranium spheres (which they called ‘briquettes‘). A second team was pressing the uranium oxide powder into spheres using a large hydraulic press (pseudo-spheres of 8.35 cm diameter). The press used had a force of 150 to 175 tons. By October 15, 1942 210 tons of graphite had been machined, and on November 16, 1942 they opened the rubberised balloon cloth envelop and started to build the pile. Work continued 24/7 by shifts. The pile itself was built within a wooden frame, and each day they segregated the better quality graphite from the lower quality. Fermi would calculate each day where to put the different grades of graphite (remember no computers, everything was calculated by hand). As they built the pile layer by layer they had also to decide where to put the uranium oxide and the uranium metal. An to top it all, more uranium metal arrived which made them adjust the core configuration on-the-fly. Then there was the problem of the location of the cadmium control strips. These control ‘rods’ were in fact just cadmium sheets nailed to a flat wooden strip to be inserted in a slot machined into the graphite. They made sure the pile remained sub-critical, the control rods were inserted as the pile was constructed, and locked in place with a padlock. One additional control rod was made to drop in by gravity, it was called the ‘zip’. The idea was that it would be suspended by a cord over the pile, a cord that could be cut in an emergency.
In all 2,060 uranium metal cylinders (5.7 cm long) were made, 14,840 uranium dioxide spheres, 1,200 triuranium octoxide spheres, 540 uranium dioxide cylinders and 840 triuranium octoxide cylinders, for a total of 46.5 tons. When they talked of spheres or pseudo-spheres what that meant was a cylinder of equal diameter and height but with the edges cut off to make them roughly spherical.
As the layers of the core were built up, reactivity measurements were made for each layer. They were able to predict that the pile would go critical on the 57th layer, during the night of December 1, 1942. During that evening the last measurement was made, and it was clear that with the removal of the last cadmium rod the pile would go critical.
The next morning the last cadmium rod was pulled step by step. The neutron activity was measured, and compared with the previous measurement and with the predicted value. Everything went according to plan, and with the complete removal of the last cadmium rod the pile went critical, and the worlds first self-sustaining chain reaction took place. Some 40-odd people were present, including Anderson, Compton, Fermi, Szilárd, Wigner,…., and also Crawford Hallock Greenewalt of Du Pont, who wanted to know that what they had contracted to build actually worked. The pile worked for 28 minutes, with an energy production of less than a watt.
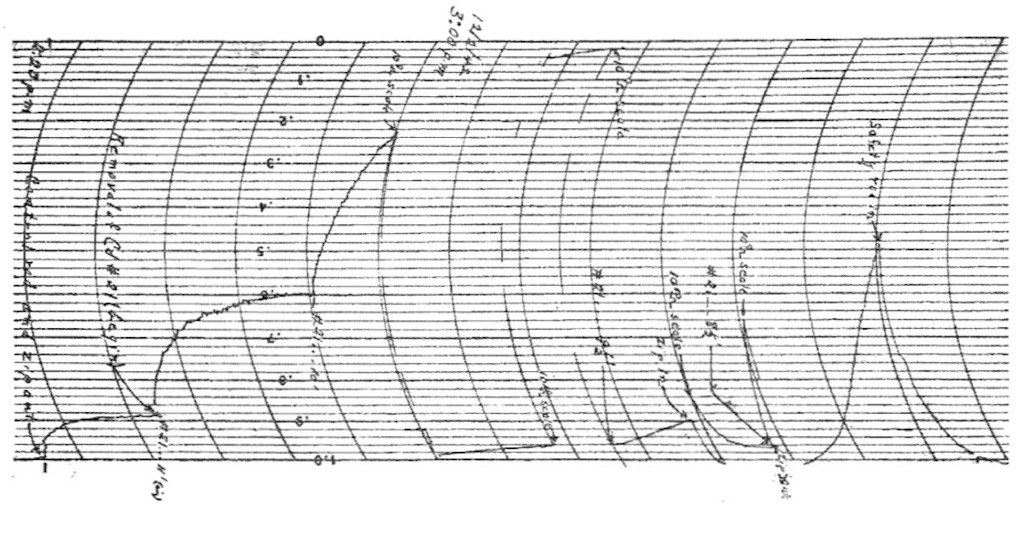
Above we have the automatic intensity recording for the first operation of the pile. We can see the exponential rise of the intensity. What we see is neutron intensity increasing from bottom to top, and time along the horizontal axis. The first thing we see on the left is the removal of the control rods. Then you see the levelling of the neutron intensity indicating that the pile is not yet critical. The big drop at about the 6th interval from the left was simply a change of scale. On the far right you can see a sharp rise to a peak and a sharp drop. This is the signal of the self-sustaining reaction, a signal that shows no sign of levelling off. The sharp drop in the signal at the end was where the control rods were reinserted in the core.
We see mentioned that Greenewalt of Du Pont was present during the Fermi’s first demonstration of a self-sustaining chain reaction. In fact the story is far more complex. With the arrival of ‘big-industry’ with Du Pont (electro-magnetic, pile and isotope separation) and Kellogg (gaseous-diffusion) Groves decided to re-appraise the entire Manhattan Project. He turned to Warren Kendall Lewis to head the review. The Committee visited Columbia for the gaseous-diffusion work. The review was positive in that they felt that the barrier was similar to a catalyst in chemical engineering, and the process looked like something that could be perfected in the chemical industry. On the other hand they did not think that the partnership Kellogg-Columbia could do the job. They then went to Chicago. The Du Pont people had visited the site some 3 week earlier, but for Lewis it was new. They listened to the presentations, but there was nothing to see (Fermi had not yet demonstrate a chain reaction). They then left for Berkeley to look at the electro-magnetic process. They saw the calutrons but remained unconvinced. They then took the train back to Chicago to see the demonstration of Fermi of the first self-sustaining chain reaction. That is why Greenewalt was there, and why he returned to the other member of the Lewis committee with “the glow of success on his face”. Even without this demonstration, the Lewis committee had already recommended to continue the work on the pile. They also recommended to build a full-scale gaseous-diffusion plant and to push forward with the calutron (although they felt that a full-sized plant with 22,000 calutrons was not practical). They thought that a pilot with 100 calutron’s could produce uranium-235 for use in physical measurements, but they could not see beyond a 100-gram plant.
On the December 12, 1942 the pile was tested again but this time at a power production of about 200 watts for 45 minutes.
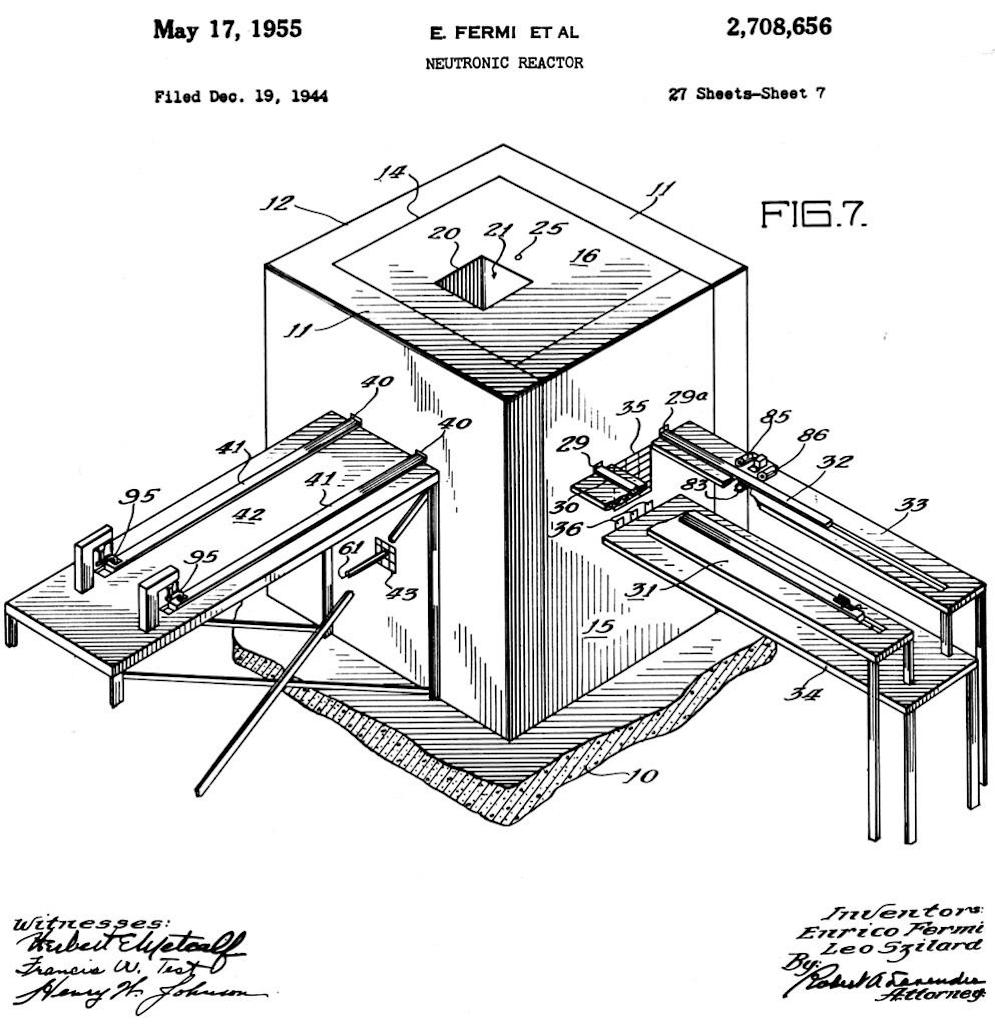
Above we have one of the diagrams from the U.S. patent held by Fermi and Szilàrd for a ‘Neutronic Reactor’, i.e. the construction and operational of self-sustaining neutron chain reacting systems with the production of power in the form of heat. What we see above is a reactor with a heavy concrete foundation (10) with side walls (11) and connected back wall (12). A vault space (14) is created in which a chain reacting lattice of uranium and graphite is erected until the vast is filled within about 2 meters from the top and from the front. The front is then completed with a concrete wall (15), and top (16) is added made of wood and lead layers. A hole in the top (20) leads to a well (21) extending inwards to the peripheral layer of uranium bodies in the internal lattice. A small shaft (25) leads to the central position of the reactor. The front wall (15) is pierced by ‘shim’ and regulating rod apertures (29 and 29a). A ‘shim’ or limiting rod (30) is positioned on a platform (31) and is moveable, and a control rod (32) is positioned in a similar way. Below there is a removable platform (34) that can receive lattice portions that can be removed from the reactor through a channel (35) and from the stringer channels (36). Two safety rods (41) can be inserted through apertures (40). Below there is an ionisation chamber channel (43).
The self-sustaining chain reaction occurs by building up within the space (14) uranium metal and oxide and graphite blocks, creating a ‘pile’. Certain graphite blocs have holes for the uranium bodies (‘live’ graphite), those that do not have holes are ‘dead’ graphite. The uranium bodies are placed in the holes in the live graphite, and are used to build up the chain reacting system. As the reactor is built layer by layer the ionisation chamber, the shims, the regulating rods and the safety rods are added. The patent describes the experimental operation of the reactor as well as the design of the components, including the fuel, graphite and different rods inserted through the pile.
Fermi’s co-author on may papers, H. L. Anderson, produced a wonderful observation on Fermi and on the newly constructed pile. The pile promised atomic energy and for some atomic bombs. But it also promised something new and unexpected. The pile because a fantastic experimental tool. Fermi noted that experiments that were almost impossible to perform before, became easy beyond his wildest dreams. The pile was a kind of neutron multiplier. Very minor changes to the neutron population were multiplied a million times. For example the temperature coefficient of the multiplication factor, long a difficult problem, could now be measured with great precision. The pile was sensitive to some cold air entering the room through an open window. The pile was an excellent device to check the purity of uranium, and for the study of the effects of really minor changes to the lattice design, etc.
Finally the original West Stand pile, named Chicago Pile 1 (CP-1) had a short life, and after three months it was taken apart and replaced by CP-2 built at Argonne Forest. The focus of the work changed, and CP-2 and later piles were important in connection with the design of the Hanford plant (site W). Uranium metal was tested, control rods were designed and tested, shielding was tested, the presence of water in the pile was studied, etc. By October-November 1943 pile engineering had been more or completely transferred to Oak Ridge (some times known as Site X or Clinton Engineering Works). In addition they had a working pile that was producing small quantities of plutonium sufficient to test chemical separation.

The impression given is one of am experiment rapidly thrown together in a dingy corner of an old football stadium. There are no photographs of that moment, but above we can see that CP-3 built at Argonne Forest was far from some ‘Lego’ set up in a corner somewhere.
By April 1944 more and more people from the Chicago team were working in Hanford, and Fermi himself was increasingly being called to Los Alamos (he moved there in September 1944 but moved back to Chicago December 31, 1945).
Nuclear Physics - Simplified
At one point in time Fermi tried to explain what was planned using ‘simple’ language. He talked about the physics of the pile, and the meaning of the multiplication factor ƙ (which Fermi always called the reproduction factor). Fermi’s definition of ƙ was the “number by which one multiplies the number of neutrons of one generation to find the number of neutrons in the next generation“. So if you have Ni neutrons in generation i there will be ƙNi daughter neutrons and there will be ƙ2Ni neutrons in the 2nd generation, and ƙnNi neutrons in the nth generation. If ƙ<1, then ƙnNi will tend to 0, and the reaction is not self-sustaining. If ƙ=1, then the reaction is self-sustaining, and if ƙ>>1, then “run as fast as you can and get behind a big hill miles away“.
Fermi went on to explain in a little more detail the life of a neutron. A neutron is born in a uranium lump with a kinetic energy of 2 MeV, and before it escapes the lump it can collide elastically with a uranium atom, transferring a small part of its kinetic energy to the translational motion of the nucleus but leaving the state of the nucleus unchanged. The neutron will travel on with a slight loss in kinetic energy. The neutron can also collide inelastically with a uranium nucleus, it can escape but it imparts a larger part of its kinetic energy to excite the nucleus to a higher energy state. The nucleus may then, for example, emit a gamma-ray. The neutron can also collide with a nucleus and caused fission. In this case the neutron is captured. Fission in uranium-235 can be caused by both fast and slow neutrons. To cause fission in uranium-238 the neutron must have an energy greater than 1 MeV. The chance of this happening for a 2 MeV neutron born deep within the lump is about 15%. In general when fission occurs the incident neutron disappears and a little less than 2.6 neutrons appear, i.e. a multiplication factor of about 1.6 (average multiplication factor per neutron for the process of fast fission in natural uranium metal).
However, most of the neutrons actually escape the uranium metal lump because they are born nearer the surface. This is in part due to the fact that they are created from fissions induced by thermal neutrons which come in from the graphite and die near the surface of the uranium metal lump. Thermal neutrons have a high probability to be absorbed in the surface of the lump.
As neutrons slow they may be caught in the resonance bonds of the metal uranium. This resonance absorption is a function of the volume of the uranium metal lump. If the neutron has the energy corresponding to a resonance absorption peak it is almost immediately absorbed as it hits the lump, and thus the number of neutrons absorbed is proportional to the surface of the lump. If the neutron energy does not correspond to a high absorption coefficient it may travel some distance into the lump before finally being absorbed, so the number of neutron absorbed by a lump is proportional to the mass of the lump.
The way the pile was designed the idea is that about 0.887 of the fast neutrons will escape resonance absorption and will thus reach thermal energies. For every thermal neutron produced, only 0.868 thermal neutrons are re-absorbed by the uranium metal, and for every thermal neutron absorbed, 1.29 neutrons will be produced. From this 0.887 x 0.868 x 1.29 = 0.993 is the fraction of new neutrons from slow fission per original fast neutron. Correcting for the contribution of fissions by fast neutrons, yields the multiplication factor ƙ, which in theory should be 1.086 (the average number of neutrons produced by one neutron).
What Fermi planned was to create a self-sustaining chain reaction, with the initial neutrons provided by spontaneous fissions in the pile. The energy of these first neutrons will be reduced so that when they finally come into contact with ‘special materials’ new neutrons are born very readily. A fraction of this new generation of neutrons then repeat the life history of their parents.
The pile will be a big cube of graphite 6.4 metres on each side, and consisting of carbon blocks and uranium units. The uranium will produce neutrons and the carbon blocks will slow them down (or ‘moderate’ them).
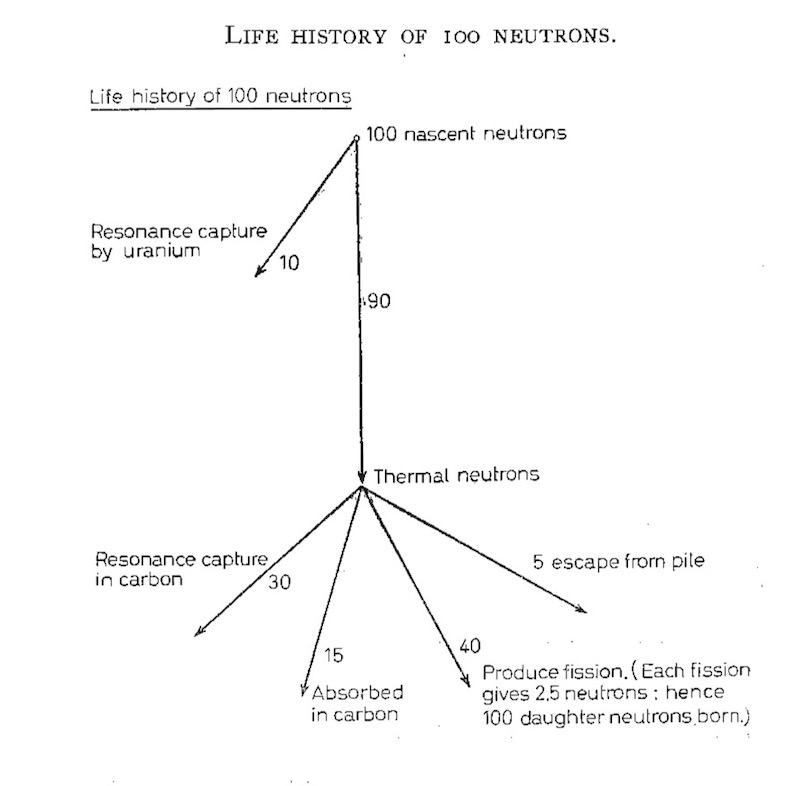
There are three things that can happen to the neutrons (the above diagram comes from one of Fermi’s reports). Firstly, neutrons can escape, and to reduce this the pile has been made very big. Secondly, neutrons can be simply captured and disappear. Thirdly, they can produce a fission and release daughter neutrons. The geometry of the pile is designed to reduce the second probability and increase the third.
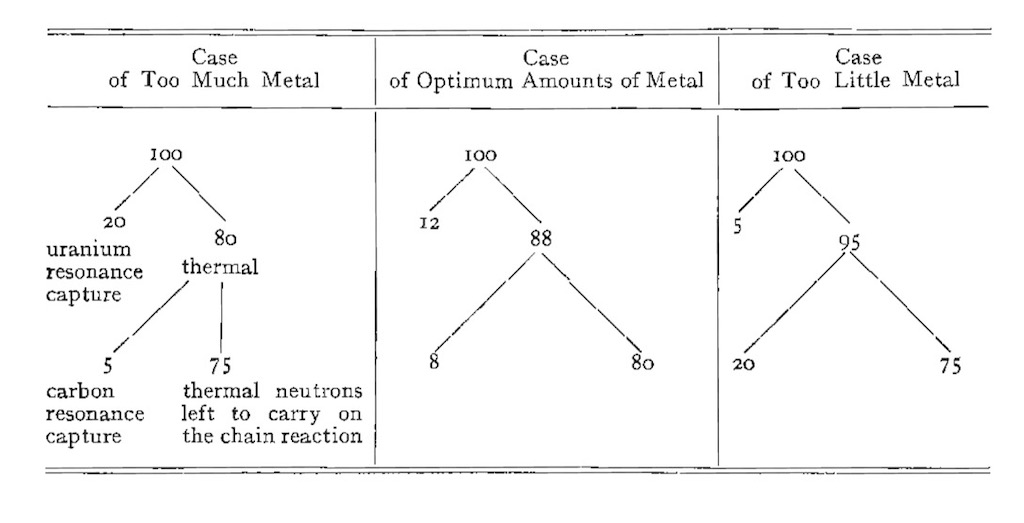
Again taken from one of Fermi’s original reports he describes why a particular lattice structure was chosen. When Fermi mentions ‘metal’ it means uranium metal. The key is to manage the number of neutrons lost by thermal and fast resonance absorption, these are lumped together in the single figures 20, 12, and 5. Some neutrons will die in the uranium and some in the carbon, both will be lost to the chain reaction. It there is too little metal more neutrons will die by uranium resonance absorption, but if the number of neutrons lost by carbon and uranium resonance absorption is minimised more neutrons are left for the chain reaction.
There will be special neutron counters in the pile to measure the total number of neutrons in the pile at any time (neutron density), and ƙ will be these neutrons minus those that manage to escape from the pile. The pile will be built up with 4 rods specially designed to absorb thermal neutrons. These rods can be moved in and out horizontally and control ƙ. When the pile is complete the whole will be in a rubberised balloon cloth and will be evacuated to remove the air, reducing even more any parasitic capture. Then gradually the control robs will be pulled out and ƙ will approach 1. If and when this happens everyone will leave the area and they will try to see if power can be obtained from the pile.
Put like that it sounds simple!
Another task that Fermi took on was to brief the engineers who would build the plutonium piles at Hanford. One masterful contribution was on the idea of a self-sustaining reaction. The whole question turned about two factors. The first was the multiplication factor (reproduction factor) ƙ. A big pile (or reactor) will be ‘chain-reacting’ if ƙ>1. If ƙ<1 then it does not matter how big the reactor is. Secondly, some neutrons will be lost from a reactor of a finite size, and the key is to retain as many as possible inside the pile. The bigger the reactor the smaller the loss, for an infinite reactor the neutron loss would be 0%. Interestingly if we define something called the coefficient of retention l, then ƙl = 1. If the multiplication factor is greater than 1 by only a very small amount, the size of the chain-reacting system would have to be very large in order to minimise the loss of neutrons.
It would be great to be able to calculate an exact multiplication factor ƙ but it was found to be impossible because it was not practical to measure with precision the numerous constants involved in the equations (in 1942 the error would have been about +/-15%). Calculations pointed to a ƙ of about 1, but would it be above 1, or below 1? So the only way was to measure ƙ as precisely as possible, and the important thing was that different configurations resulted in the same ƙ to within 1%. During the experiments what emerged was that the materials used must be of an exceedingly high purity, particularly free of boron and several rare earth elements. During the experiments a ƙ=1.04 was found, and a ƙ=1.07 was predicted for uranium metal. The experiments were not full sized piles, so there remained the question about the precise amount of materials that would be required for a chain-reacting system. Knowing ƙ to within 1% just meant that they knew the amount of materials needed to +/-40%. For a metal-graphite system the error would still have been +/-15%.
Another problem with a full-sized system was how the reactivity of the system might vary with temperature. If the temperature rose with a rise in reactivity, then an accidental increase in temperature could have be very dangerous. If the reactivity decreased with increasing temperature, then the system would be thermally stable. Even in 1942 the tests performed showed that this second situation was the most likely, but the small changes they were measuring were about the same as the experimental error! At the time of the experiment on a full-size pile this question was still outstanding. It was for this reason that a controlling mechanism was needed so that the reaction could be interrupted.
The thermal stability of the system was not guaranteed, therefore a control system was installed. The key was to use a material that had strong neutron absorption characteristics. The idea was to have solid rods that could be pushed mechanically in to slots in the pile. An alternative might have been to use a liquid that would fill up pipes inside the pile. A gas might also have done this if it were introduced under pressure and filled all the empty spaces. The option of solid rods was retained, but it was predicted that in a power producing reactor they would also need to be cooled to avoid warping of the rods.
In addition to the control rods, a separate set of rods should be kept as safety rods. These rods should be designed to work in all conditions, including should they warp. Fermi felt that an additional back-up might be needed, and he suggested the possibility to flood the system with a neutron absorbing liquid.
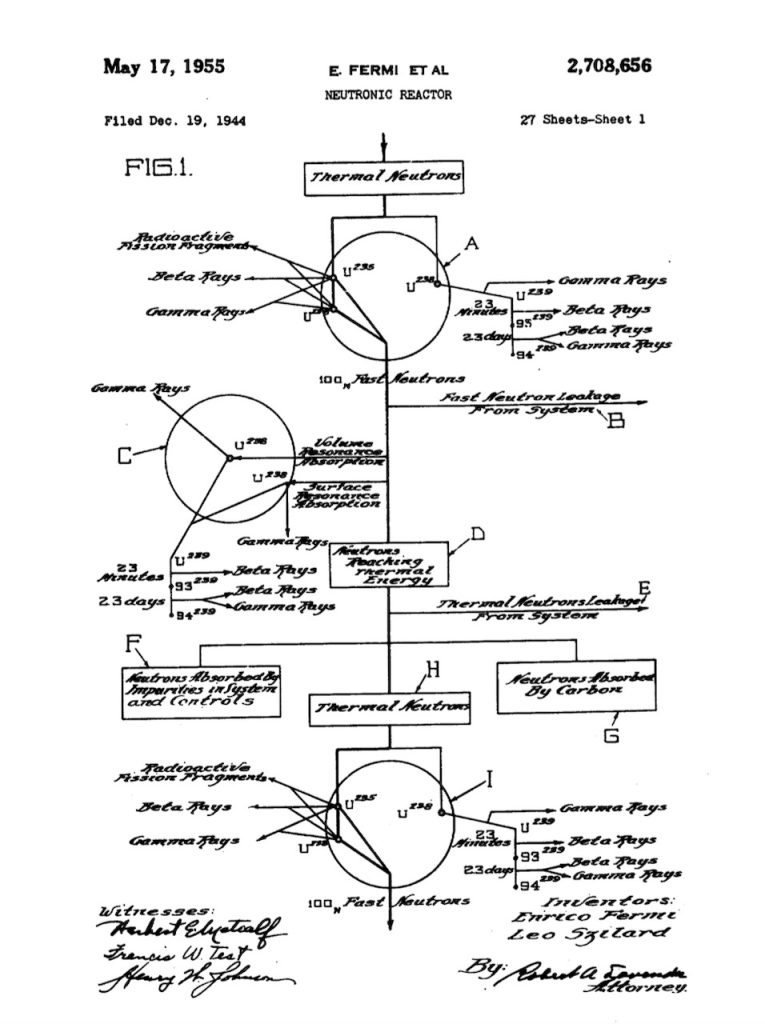
As an extension of this section on ‘simplified nuclear physics’ I have added below the description used by Fermi and Szilàrd in their U.S. patent for a ‘Nuctronic Reactor’. It describes the chain reaction process, as it was known at the time. What the authors described was a finite system using natural uranium bodies or lumps dispersed in a graphite moderator within a larger reactor body where the neutron density is substantially constant. I am using the language and terminology from the patent file.
A represents fast neutrons being set free as a result of a fission process.
B represents a fast neutron loss due to leakage out of the system.
C represents a uranium body of any size in which both volume and surface resonance absorption of neutrons by uranium-238 takes place, at resonance energies above the thermal energy, and leading to the formation of element-94.
D represents the number of neutrons reaching thermal energy.
E represents a thermal neutron loss by diffusion out of the system.
F represents a neutron loss caused by capture of neutrons by impurities in uranium, graphite, and controls.
G represents a neutron loss due to capture of thermal neutrons by the graphite as the thermal neutrons diffuse before emerging uranium.
H represents the number of thermal neutrons entering a uranium body.
I represents a uranium body of any size in which part of the thermal neutrons entering the body are absorbed by uranium-238 leading to the formation of plutonium-239, the remaining thermal neutrons causing new fissions in uranium-238 thereby producing fast neutrons, a few of which produce additional fast neutrons by fission of uranium-238 atoms in the same body.
Some of the thermal neutrons entering uranium body A will cause fission in the uranium-235 to produce fast neutrons, the yield being at an average rate of about 2 neutrons per fission. As a result of this fission, fission fragments are released together with beta and gamma rays, producing energy which, in the system, is manifested mostly by the heating of the uranium bodies with only a slight release of heat to the graphite. The actual average yield of fast neutrons by fission of uranium-235 is slightly higher, by a few percent above the average of 2 mentioned above. Some of the fast neutrons released in the fission of uranium-235 by thermal neutrons almost immediately produce fast fission of uranium-238 in the same uranium body, with the production of additional fast neutrons.
The fast neutrons leaving the uranium body enter the mass of moderator and travel with paths that are long in comparison with the spacing of the uranium bodies. These neutrons will undergo successive collisions that slow them down. A substantial proportion of the fast neutrons are thus destined to be reduced, by about 100 elastic collisions apiece in the case of graphite and mostly in the moderator, to thermal energy. During this travel, before the neutrons arrive at thermal energies, a small percentage of the higher energy neutrons on the average may leak out of the system because of the finite size of the reactor, and be lost to the chain reaction. Furthermore, during the extremely irregular path of the neutrons while they are being slowed down by elastic collisions in the graphite, some of the neutrons will reach a uranium resonance absorption energy as they are about to enter a uranium body (such as C), and are absorbed immediately on or close to the surface of the uranium body. In addition some neutrons are reduced to resonance energy after entering the uranium body by an elastic collision with the uranium, and are immediately absorbed within the uranium body. Irrespective of whether the neutron resource adsorption in uranium-238 is on the surface, or in the volume of the uranium body, element-94 is produced by the resonance absorption according to

A small amount of plutonium-240 may also be found, formed by addition of a neutron to plutonium-239. Capture of thermal neutrons by uranium-238 as indicated in bodes A and C, also results in production of element-94 by the same process.
The predominant isotope produced, plutonium-239, is a long-lived radioactive product with a half-life of about 20,000 years.
A large percentage of the original fast neutrons escape resonance capture and fast neutron leakage, and are reduced to thermal energy within the system. Of these thermal neutrons, a small number on the average may leak by diffusion out of the system and be lost from the chain reaction, leaving the remainder of the thermal neutrons diffusing through the moderator in condition to produce fission if they promptly enter uranium-235 or element-94 without being captured by any other material.
The fission reaction is:-
uranium-235 + neutron = A + B + n neutrons (on average)
A = ‘light’ fission fragment, e.g. Br, Kr, Rb, Sr, Y, Zr, Cb, Mo, Ru, Rh. Atomic Mass 83-99 inclusive, or Atomic Number 35-45 inclusive.
B = ‘heavy’ fission fragment, e.g. Sb, Te, I, Xe, Cs, Ba, La, Ce, Pr, Nd. Atomic Mass 127-141 inclusive, or Atomic Number 51-60 inclusive.
In any practical system, impurities will be present in both the moderator and the uranium. In the chain described, a small fraction of neutrons can be captured and absorbed by impurities in the system without the reproduction factor of the system falling below unity. Also many of the thermal neutrons diffusing through the moderator are not in a position to promptly enter a uranium mass when they reach thermal energy, these thermal neutrons must continue to diffuse through the moderator until they do reach a uranium body. During this diffusion, a small percentage of the neutrons are absorbed by the moderator, leaving sufficient thermal neutrons to enter a uranium body to produce new fast neutrons by fission, to repeat the cycle. In a uranium-graphite system for every 100 fast neutrons about 72 thermal neutrons enter the uranium body to produce 100 new fast neutrons, i.e. a survival of about 72% of the original 100 fast neutrons during the slowing process.
The four neutron losses from the chain reaction referred to in the above figure, where the resonance absorption at C and the friction of thermal neutrons absorbed by uranium-238 at I represent the uranium absorption losses. Losses due to impurities are represented at F, those due to absorption in the moderator at G, and the leakage losses due to the finite size of the system at B and E.
It is possible to substantially reduce uranium resonance absorption by using light elements for moderators, few collisions are required to slow the neutrons to thermal energies with larger increments of energy loss per collision, thus decreasing the probability of a neutron being at a resonance energy as it enters a uranium atom. During the moderation, however, neutrons are moving through the slowing medium over random paths and distances so that the uranium is not only exposed to thermal neutrons but also to neutrons of energies varying between the energy of fission and thermal energy. Neutrons at uranium resonance energies will, if they enter uranium at these energies, be absorbed on the surface of a uranium body whatever its size, giving rise to surface absorption. And substantial reduction of overall surface of the same amount of uranium will reduce surface absorption, and any such reduction in surface absorption will release neutrons to enter directly into the chain reaction.
Neutrons are also subject to capture by the moderator. While carbon and beryllium have very small capture cross sections for thermal neutrons, and deuterium still smaller, a fraction of the thermal neutrons present in the system even under best conditions is lost by capture in the moderator during diffusion. It is desirable to have the neutrons reaching thermal energy enter uranium as promptly as possible. This may be taken care of by using optimum or near optimum geometry where the resonance absorption is substantially equal to absorption in the moderator.
However, even when resonance and moderator losses are educed to a practical minimum, no self-sustaining chain reaction can be obtained in any system unless impurities in the materials used for the reaction are reduced to such an extent that the loss by parasitic capture, together with other losses, prevent the reaction from becoming self-sustaining. Impurities present in both the uranium and the moderator consequently constitute a very important neutron loss factor in the chain. The effectiveness of various elements as neutron absorbers varies tremendously.
In any chain reacting system, it is only when the system has infinite size the there will be no exterior leakage of neutrons from the system. For any reactor of finite size exterior neutron losses will occur, and these losses will increase as the size of the reactor decreases.